[Solved] There is an m x n matrix that is initialized to all 0’s. There is also a 2D array indices where each indices[i] = [ri, ci] represents a 0-indexed location to perform some increment operations on the matrix.

Question

There is an m x n matrix that is initialized to all 0‘s. There is also a 2D array indices where each indices[i] = [ri, ci] represents a 0-indexed location to perform some increment operations on the matrix.

For each location indices[i], do both of the following:

1. Increment all the cells on row ri.
2. Increment all the cells on column ci.

Given m, n, and indices, return the number of odd-valued cells in the matrix after applying the increment to all locations in indices.

Example 1:

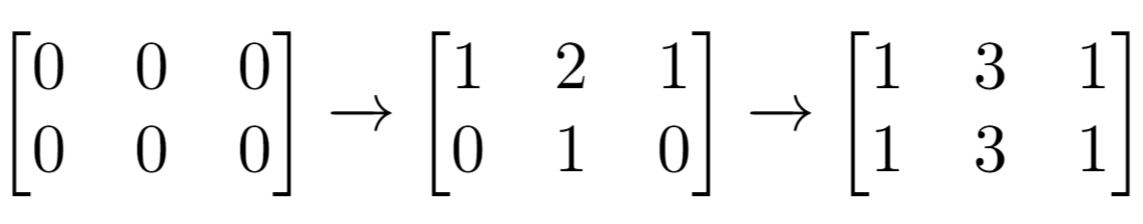
Input: m = 2, n = 3, indices = [[0,1],[1,1]]
Output: 6
Explanation: Initial matrix = [[0,0,0],[0,0,0]].
After applying first increment it becomes [[1,2,1],[0,1,0]].
The final matrix is [[1,3,1],[1,3,1]], which contains 6 odd numbers.

Example 2:

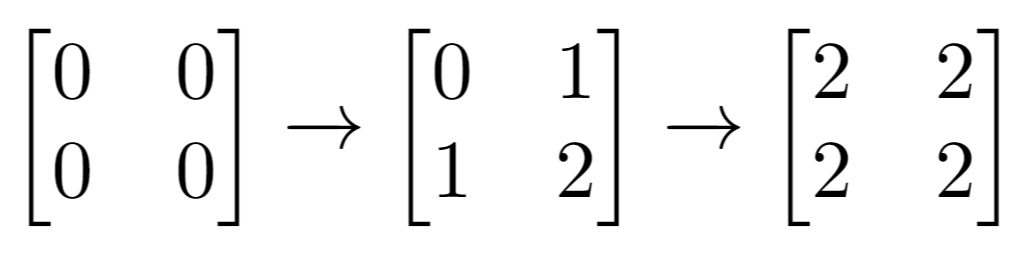
Input: m = 2, n = 2, indices = [[1,1],[0,0]]
Output: 0
Explanation: Final matrix = [[2,2],[2,2]]. There are no odd numbers in the final matrix.

Constraints:

• 1 <= m, n <= 50
• 1 <= indices.length <= 100
• 0 <= ri < m
• 0 <= ci < n

Follow up: Could you solve this in O(n + m + indices.length) time with only O(n + m) extra space?

Python Solution

class Solution:
    def oddCells(self, n: int, m: int, indices: List[List[int]]) -> int:
        rows = [0]*n
        cols = [0]*m

        for i,j in indices:
            rows[i] = rows[i]^1
            cols[j] = cols[j]^1

        c=0
        for i in range(len(rows)):
            for j in range(len(cols)):
                if rows[i]^cols[j]==1:
                    c+=1
        return c